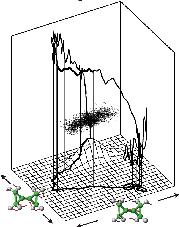
Inhibition Studies on hAQP1
Conformational Flooding
Chemical Flooding
Predicting Unimolecular Chemical Reactions: Chemical Flooding
The calculation of reaction pathways, energy barriers and reaction rates for chemical reactions of small molecules is a routine task in todays theoretical quantum chemistry. Established methods exist for tasks like (a) minimization of educt states, (b) exploring potential energy hypersurfaces, (c) detecting transition and product states, (d) finding reaction pathways connecting the educt, transition and product state, and (e) calculating the reaction rates by associated quantum statistical mechanical approaches.
Assuming a given educt state, all these methods require additional knowledge on the reaction pathway, putative transition states or the product state.
Recently, a force field based method, 'conformational flooding'[4] has been developed to predict structural transitions of macromolecules. Here we extend that method towards the calculation of chemical reactions using density functional theory. Accordingly, that extension, called 'chemical flooding', should enable one to predict in an unbiased manner reaction pathways, transition states and accessible product states for dissociation reactions of small molecules. 'Chemical Flooding' also provides estimates for activation energies, which can and should subsequently be refined with already established methods.
|
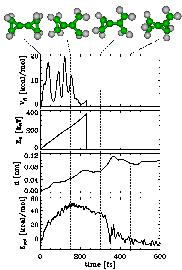
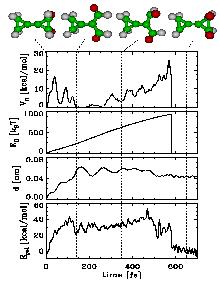
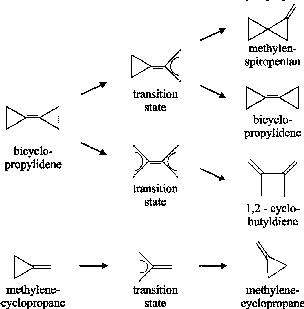
As a test case, we studied the known dissociation reaction of two molecules, bicyclopropylidene (BCP) and methylenecyclopropane (MCP)[1].
Results
References:
[1] A. de Meijere, S. Kozhushkov, and A. F. Khlebnikov. Topics in Current Chemistry , Vol. 207, Springer-Verlag, Berlin, Heidelberg, 2000.
[2] W. R. Dolbier, K. Akiba, J. M. Riemann, C. A. Harmon, M. Bertrand, A. Bezaguet, and M. Santelli, J. Am. Chem. Soc., 93(16):3933-3940, 1971
[3] M.Eichinger, H. Grubmüller and H.Heller. User Manual for {EGO\_VIII}, Release 1.0, 1995, electronic access: EGO-Manual
[4] H. Grubmüller, Phys. Rev. E52 (1995),2893
An HTML-version
and a
postscript-version
are available.
[5] J. Hutter et al. CPMD version 3.0 manual. IBM Research Division, Zürich research Lab. MPI für Festkörperforschung, Stuttgart, 1995-1998